The Making of Musical Cosmos Maps - part 1
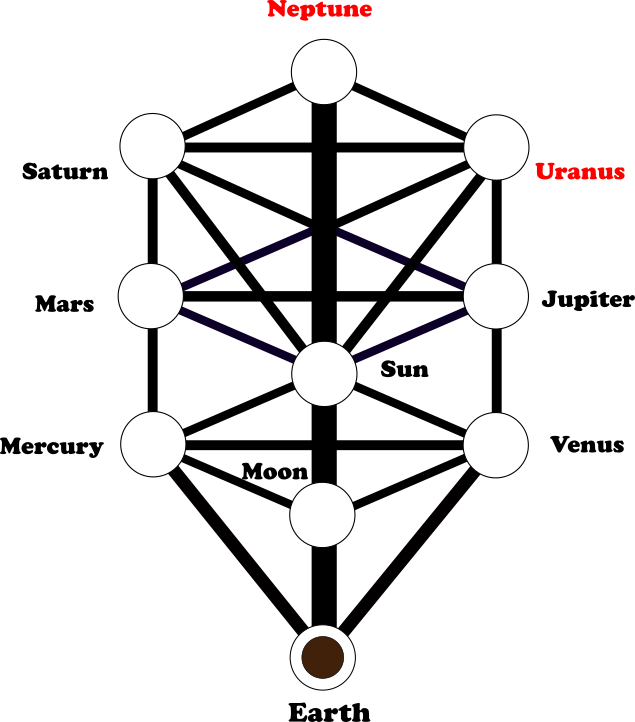
Figure 1. The 2nd Harmonic in the Solar System. This is a very wide spread and common Harmonic in other star systems ( if our current data is accurate ) as well as our own. The second harmonic in the map above means that there are points in the orbit of the planets in question where they are twice or half the distance from the Sun as another planet or body. Essentially it means that all these planets play the same note at some point in their orbit. I call this pattern above, the Planetary Octave, though it doesn’t precisely mean that they all play the same note. Even though there is a planetary octave pattern with this shape, in reality it comes in a few forms if it is to be the same note. From Jupiter to Eris is one note (excluding Neptune), with Jupiter starting the pattern at 750 million km. From there all the outer bodies align to play the same note. Similarly if you start with Ceres at 432 million km, you have the same note from Ceres to Mercury (excluding the Earth). In this way, the Planetary octave is made of 2 notes. The other discrepancy worth noting is that Neptune is only included here because Eris resonates with all nearby bodies on the diagram but even from the diagram above you can see that Neptune isn’t included in the planetary octave in the same way as the others—Neptune is skipped from Uranus to Pluto.
Here is a quick run through of the construction of musical cosmos maps. I want to keep this as clear and simple as possible, so that even if you don't understand music theory you can still understand how these maps were made and some of the principles involved. This is a run through of the ideas and algorithms involved in re-creating the tree of life from NASA’s planetary data and mapping languages to the paths on these Musical Cosmos diagrams.
How to create a basic musical map for a solar system
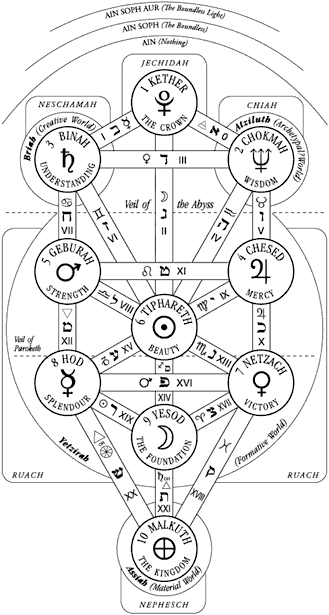
First I started out with an old style of map making used by mystics to describe energy and the solar system: the Hermetic and Hebrew diagram called the Tree of Life.
Figure 2. An example of a Traditional Hebrew Tree of Life from Joseph Gikatilla's Gates of Light.
I use a recreated map of the tree of life which is organized by Harmonics, as seen at the top of the article. If it isn't the traditional map it still gives you a measure of how the physical world connects to other hermetic and qabalistic diagrams that are more traditional, like the diagrams below.
HARMONICS
There are only a handful of musical ideas one needs to construct this style of musical cosmos map depicted at the top of the article. With a clear idea of Harmonics alone, most of the maps can be constructed quite simply. Though some maps are constructed with other harmonics the basic idea of harmonics can be understood as simple math. So if you can multiply or divide you can understand harmonics, because they are the same thing.
All harmonics are organized by the idea of simple multiplication or division. If you have a potent electric current or a really loud sound, these Harmonics come into play. To find them you simply multiply (for overtones) or divide (for undertones) the frequency involved by all the integers in succession- 1,2,3,4,5,6,7,8…—to identify the frequencies which are harmonically resonant with any frequency in question.
The interesting part is that all the integers reduce to very specific musical notes in relation to any original note. But when does a frequency become a musical note? The Harmonics can be thought of as a definition of music, as much as rules of thumb for patterns that exist in nature as regards energy and resonance. Each Harmonic has a character, though they are all repeated at some point on down or up the chain of simple integer numbers by division and multiplication.
Defining the Harmonics of the simplest version Tree of Life
To get the 1st Harmonic (Figure 3 below) you simply multiply or divide any frequency by 1. This is called the fundamental frequency or prime harmony. Any frequency of vibration becomes a note with the first Harmonic, as any frequency times 1 is just the same old frequency itself. So all frequencies of vibration can be construed as musical notes by this very same principle.
Figure 3. The First Harmonic in the Solar System. In this version the diagram has a line between any 2 bodies that are the same distance from the Sun at some point in their orbit. Here there are 2 lines for the First Harmonic— (1) Pluto to Neptune, and (2) Pluto to Eris. It is termed orbital war cry because it means there is a possibility of a collision baked into their orbital paths.
The 2nd Harmonic (Figure 1) is any frequency of vibration multiplied or divided by 2, also called Octave Harmony. In music, the second Harmonic shows you all the frequencies of vibration that are regarded as the same as the first Harmonic. This Harmonic is the essential first step on the musical spiral in that it tells you what frequencies are regarded as the same or in the same family. In some ways the depth of this Harmonic is so profound that it might take some time to sink in.
The second Harmonic gives you one of the most important principles of western music: the Octave.
What it means is that any frequency has an endless number of 'siblings' that are regarded as the same musical note even though they are also different frequencies of vibration. Every time you multiply something by 2 you get a higher octave resonance of the same note. It is not really just in sound that this applies. This is also an important part of energy and especially powerful energetic currents and energy fields.
The second Harmonic is 2 times or half as much as the 'original frequency'. But it is also important to note that any frequency can take on this pattern as a part of how it resonates with other frequencies of vibration.
The 3rd Harmonic is 3 times or a third of any frequency of vibration. The third Harmonic is very close to what is called a perfect fifth in western music. Even in other forms of music on Earth, the perfect fifth is very popular, especially as it is essential to the pentatonic scale which is used in many cultures. In the Tree of Life diagram we are using here, this Harmonic is responsible for the middle Pillar, which includes the Sun, Earth, Neptune, and Eris. The diagram below shows you the third Harmonic via the planetary distances from the Sun.
Figure 4. The 3rd Harmonic of the Solar System. This I have termed Integrative Opposition because it is close to a perfect fifth musically speaking. Also it is a point just beyond the opposite point of the root note on a logarithmic musical spiral. The opposite point or halfway point for western music is equal to the square root of 2 or roughly 1.4140… This is strange in some ways to think of 1.41 as halfway between 1 and 2, but it is the logarithmic halfway point, not the linear halfway point which the perfect fifth defines. In some ways, this is perhaps why the perfect fifth is so pleasant—because it is an interesting intersection point between the logarithmic world and the linear world.
The biggest discrepancy between the 3rd Harmonic and the map above is that it is really just the two bodies, Earth and Neptune, that fit the third Harmonic more than the 2nd and 4th Harmonics (which forms the side Pillars and the Lightening Flash) . The Sun is the point from which all the others are measured, so it is not really in this group any more than the other two previous Harmonics because it is the Origin point to which all the others are compared to in order to arrive at the patterns in this map. The Sun is a part of all of these Harmonics in some ways and also is not included in any of them in other ways because it is the origin point here.
Eris is included in the middle Pillar mostly because her orbit is the only one which covers the whole musical spectrum in her orbit. This is because Eris has a very elliptical orbit compared to most of the other planets. What it means is that Eris functions as a musical integrator for all bodies in the solar system to a degree. The planets that have this sort of elliptical orbit in our solar system are the smallest ones. The largest planets in our system have very circular orbital paths, which means they are musically quite partial to only a few notes, whereas Eris plays every possible note in her orbit, though she is very tiny compared to Jupiter. She is included in the middle Pillar also to give a more symmetrical quality to the Sun, and this holds pretty well since Eris is also musical to all others because of her orbit–in a different manner than how the Sun is included in all calculations but it is also quite analogous.
The 4th Harmonic is 4 times or a fourth of any frequency of vibration. It is also a repetition of the second Harmonic to a degree simply because it is also the same thing— since 2 times 2 equals four, the 4th Harmonic also yields the next octave. In the diagram below the Fourth Harmonic lends the Pillar structure on the sides. In Hermetic Qabalah they are called the pillars of Severity and Mercy. Here they are a bit different than is normally depicted because there are more points of interest than in the traditional map.
The main difference between Musical Cosmos Maps and the Traditional Tree of Life occurs between Mars and Jupiter—Ceres is added because she fills in a very important gap in the planetary octave of the older diagram.
Figure 5. The 4th Harmonic of the Solar System.
PATHS and Language
When I was constructing these diagrams and maps one of my intentions was to bring this pattern to the world stage instead of a few select folks who know Hebrew. So in this way the question of the Paths and how they might be constructed in a way that promotes cultural exchange instead of cultural dominance was at the forefront of the project.
So I looked at how the Tree of Life can be used to hold any language as a valuable and magical thing that connects you to the stars and their power. But how could I go about it in such a way that my own limitations as a linguist would not hamper my own understanding of which letters would go where. Also if I used some sort of personal associative strategy, like "this letter means this to me, so it goes here", then it wouldn't necessarily have any ready meaning for anyone else.
So I used an algorithm that anyone could recreate if they knew the strategy and how I got there.
Figure 4. A Musical Cosmos Map for English Qaballa.
For this map in particular I used 3 points to organize the order of the letters of the English language and the paths:
Only one path between any 2 planets
Order the assignments of the letters per path to the Harmonics first (basically counting from 1, 2, 3, 4 , 6 to 8 here)
Then the closest planets go first within each Harmonic.
For the first Harmonic (Figure 3) , or 1 : 1, we have 2 paths in this map, shown in pink: Neptune to Pluto, and Pluto to Eris.
So in this case, Neptune and Pluto get the first letter, A, because the first Harmonic is first in the order of Harmonics, and also because Neptune and Pluto are closer to the Sun than Eris. Pluto and Eris get the Next letter, B, for the same reason.
If we move on to the 2nd Harmonic, we have 10 paths that fulfill that measure of 2 : 1.
Starting with Mercury to Venus first, as they are the closest, that path is assigned the letter, C.
The map of the English Language is color coded so that each of the Harmonics are separate enough visually to fill in the letters without too much effort. The best part for me was that I did not have to debate much about what went where, nor did I bake the paths and their Harmonics such that they “made more sense to me” in some other arrangement. These maps all follow the same sort of algorithm wherever possible, in hopes that it makes their re-creation possible as long as the original principles and ideas of their construction are followed.